JelloMatrix Result

If this looks like the beginning of a new math, that is because it is. It's actually a very old math reborn.

Welcome. Contact me directly at ana at jellobrain dot com if you'd like to talk about it.

This tool takes two numbers and creates a matrix grid with them, and then performs all sorts of calculations including harmonics and derivative value shifts between numbers in their numerical contexts (topologies).

In addition and perhaps more specifically, this tool evaluates matrices spliced with inverse (upside down) copies of themselves, and looks for waveforms in the resulting numerical topologies with the following characteristics:

Bands of numbers in the spliced matrix with equal values adjacent to one another...
which connect in predictable sine wave forms with one another...
following the order of a scale which is determined by the top row of values in the unspliced and native "seed" matrix...
rhythms that are even numbered change polarity at the crests of the waveforms, while odd rhythms change polarity at each shift in position.
and harmonically cycle between zero and infinity.

Aspects of that set of characteristics will appear even if the full pattern is not present in unison.

In addition, the patterns seem to continue to contain these inherent characheristics even when the two polar grids are spliced in a way that they are offset.

Following the grid drawings will lead you through the story of how they are created, and enterring a value in the form to offset the grids will generate an offset grid.

Offset

Base frequency.

Control Set:

Doublflip it.

Here is an example of the sounds these fields can produce.

Jellomatrix by Ana Willem is licensed since 2007 under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.
Based on a work at https://www.jellobrain.com.

You have scales!

The Original Matrix

1	8	2	9	3	10	4	11	5	12	6	13	7
2	9	3	10	4	11	5	12	6	13	7	1	8
3	10	4	11	5	12	6	13	7	1	8	2	9
4	11	5	12	6	13	7	1	8	2	9	3	10
5	12	6	13	7	1	8	2	9	3	10	4	11
6	13	7	1	8	2	9	3	10	4	11	5	12
7	1	8	2	9	3	10	4	11	5	12	6	13
8	2	9	3	10	4	11	5	12	6	13	7	1
9	3	10	4	11	5	12	6	13	7	1	8	2
10	4	11	5	12	6	13	7	1	8	2	9	3
11	5	12	6	13	7	1	8	2	9	3	10	4
12	6	13	7	1	8	2	9	3	10	4	11	5
13	7	1	8	2	9	3	10	4	11	5	12	6
1	8	2	9	3	10	4	11	5	12	6	13	7
2	9	3	10	4	11	5	12	6	13	7	1	8
3	10	4	11	5	12	6	13	7	1	8	2	9
4	11	5	12	6	13	7	1	8	2	9	3	10
5	12	6	13	7	1	8	2	9	3	10	4	11
6	13	7	1	8	2	9	3	10	4	11	5	12
7	1	8	2	9	3	10	4	11	5	12	6	13

Scale Pattern:

Whether you look at each row individually, or look at each diagonal row (in forward or backward 'slash' directions) you will notice that the order of numbers is consistent on every row (or each direction of diagonal rows) and that only the starting number differs from row to row. I refer to this as a 'scale'. If the scale were to be played in a circle consisting of the numbers of the first 'tone' value, the shape formed would be the same regardless of which number you start with.

HORIZONTAL SCALE [<->] (-6/6): 1, 8, 2, 9, 3, 10, 4, 11, 5, 12, 6, 13, 7,
FORWARD SLASH DIAGONAL SCALE [/] (6/7): 1, 7, 13, 6, 12, 5, 11, 4, 10, 3, 9, 2, 8,
BACKWARD SLASH DIAGONAL SCALE [\] (8/5): 1, 9, 4, 12, 7, 2, 10, 5, 13, 8, 3, 11, 6, ...

Jellomatrix by Ana Willem is licensed since 2007 under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.
Based on a work at https://www.jellobrain.com.

The Basic Orientation of the Spliced Matrix

Why splice the initial matrix? This started out as a hunch, but also following the work of Jose Arguilles who inspired this up to a point. But also the work of Mark Rothko and Randy Powell with their ABHA torus to which the matrix forms here bare some relation but which diverge from what Randy and Mark are doing in important ways. In my mind, splicing the matrix creates an architecture that reminded me of a battery. I do not think this analogy is off-base. When we combine this notion while also looking for the patterns in the 'scales' found in the original matrix, we see emergent patterns and pathways. The next progression of images takes you through a categorization of some of those patterns.

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

HIGHLIGHTING PRIMES: The Spliced Matrix

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

HIGHLIGHTING EVEN+ODD: The Spliced Matrix

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

Interstingly enough, the sections which seem to hold information about the vortec/ies they reflect seem to fall most often in the middle of the sine waves created by what appear to be very different "environments" or "gradients" between higher frequency oscillations of even and odd numbers (you might need to squint your eyes to see them), They are the waves defined by the more or less frequent oscillatory patterns taken as a whole. More about this in the "Rows" calculations in the "Increments" section below.

HORIZONTAL SCALED WAVES

Jellomatrix by Ana Willem is licensed since 2007 under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.
Based on a work at https://www.jellobrain.com.

Prime Series of Matrix is Bi-directional

WAVE FORM POLE SHIFT: Highlighting the adjacent equal values.

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

WAVE FORM SCALES: The Waveform Scales: EVEN Rhythms

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

Scale Pattern:

This tool is meant as a proof of concept and not as a complete set of waveforms that are possible (although I am working on it!).

RED = Start of wave.

EVEN Waves

Starting 4: scale direction = reversed, rhythm = 2, initial vertical = down, color = powderblue.

Starting 4: scale direction = forward, rhythm = 6, initial vertical = down, color = orange.

Starting 4: scale direction = reversed, rhythm = 2, initial vertical = down, color = plum.

Starting 4: scale direction = forward, rhythm = 6, initial vertical = down, color = seagreen.

FORWARD BACKSLASH SCALED WAVES

Jellomatrix by Ana Willem is licensed since 2007 under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.
Based on a work at https://www.jellobrain.com.

Prime Series of Matrix is Bi-directional

FORWARD SLASH DIAGONAL SCALE [/] (6/7): 1, 7, 13, 6, 12, 5, 11, 4, 10, 3, 9, 2, 8,

WAVE FORM SCALES: The Waveform Scales: EVEN Rhythms

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

Scale Pattern:

This tool is meant as a proof of concept and not as a complete set of waveforms that are possible (although I am working on it!).

RED = Start of wave.

EVEN Waves

Starting 4: scale direction = forward, rhythm = 2, initial vertical = down, color = powderblue.

Starting 4: scale direction = reversed, rhythm = 6, initial vertical = down, color = orange.

Starting 4: scale direction = forward, rhythm = 2, initial vertical = down, color = plum.

Starting 4: scale direction = reversed, rhythm = 6, initial vertical = down, color = seagreen.

BACKWARD BACKSLASH SCALED WAVES

Jellomatrix by Ana Willem is licensed since 2007 under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.
Based on a work at https://www.jellobrain.com.

Prime Series of Matrix is Bi-directional

BACKWARD SLASH DIAGONAL SCALE [\] (8/5): 1, 9, 4, 12, 7, 2, 10, 5, 13, 8, 3, 11, 6,

WAVE FORM SCALES: The Waveform Scales: EVEN Rhythms

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

Scale Pattern:

This tool is meant as a proof of concept and not as a complete set of waveforms that are possible (although I am working on it!).

RED = Start of wave.

EVEN Waves

Starting 4: scale direction = forward, rhythm = 10, initial vertical = down, color = powderblue.

Starting 4: scale direction = forward, rhythm = 2, initial vertical = up, color = orange.

Starting 4: scale direction = forward, rhythm = 10, initial vertical = down, color = plum.

Starting 4: scale direction = forward, rhythm = 2, initial vertical = up, color = seagreen.

Jellomatrix by Ana Willem is licensed since 2007 under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.
Based on a work at https://www.jellobrain.com.

ODD/EVEN: Differences and Harmonics

These increment calculations show the relationships of the numbers in the grid by relating them to the ones in front of them (forward) and behind them (backwards) using the "tone" value as the base in the numbering system.

The diagonal increments still go down the row, but show the relationships between the number and the one diagonally above (forward) it and below it (backward).

The bold letters at the end of each row represent the Lambdona Notes that the ratios the repeating increments create.

Row

Forward (Odd/Even) (x,y)|(x+1,y)

As alluded to above, if you look at the number grid below, what I have noticed is that I can usually find 'vortex activity' starting and ending with rows that oscillate between '0' and another integer. So in this section, the vortex arrays are between "zero" and "infinity". In addition, between these rows, it seems to be important to have the intervals mirror one another as you move towards the center.

Row 1:	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	G	6+1=7
Row 2:	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	F	4+3=7
Row 3:	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	G#	2+5=7
Row 4:	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	zero	0+7=7
Row 5:	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	Eb	11+9=20
Row 6:	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	A	9+11=20
Row 7:	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	infinity	7+0=7
Row 8:	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	E	5+2=7
Row 9:	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	G	3+4=7
Row 10:	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	F	1+6=7
Row 11:	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	G	12+8=20
Row 12:	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	C	10+10=20
Row 13:	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	F	8+12=20
Row 14:	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	G	6+1=7
Row 15:	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	F	4+3=7
Row 16:	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	G#	2+5=7
Row 17:	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	zero	0+7=7
Row 18:	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	Eb	11+9=20
Row 19:	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	A	9+11=20
Row 20:	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	infinity	7+0=7

Order of frequencies: based on a 264Hz baseline or "C"

1584Hz
352Hz
105.6Hz
1.0E-7Hz
322.66666666667Hz
216Hz
10000000Hz
660Hz
198Hz
44Hz
396Hz
264Hz
176Hz
1584Hz
352Hz
105.6Hz
1.0E-7Hz
322.66666666667Hz
216Hz
10000000Hz

Backward (Odd/Even) (x,y)|(x-1,y)

Row 1:	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	Eb	7+12=19
Row 2:	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	Bb	9+10=19
Row 3:	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	F#	11+8=19
Row 4:	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	zero	0+6=6
Row 5:	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	C	2+4=6
Row 6:	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	C	4+2=6
Row 7:	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	infinity	6+0=6
Row 8:	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	F#	8+11=19
Row 9:	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	D	10+9=19
Row 10:	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	A	12+7=19
Row 11:	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	G#	1+5=6
Row 12:	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	C	3+3=6
Row 13:	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	E	5+1=6
Row 14:	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	Eb	7+12=19
Row 15:	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	Bb	9+10=19
Row 16:	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	F#	11+8=19
Row 17:	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	zero	0+6=6
Row 18:	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	C	2+4=6
Row 19:	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	C	4+2=6
Row 20:	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	infinity	6+0=6

Order of frequencies: based on a 264Hz baseline or "C"

154Hz
237.6Hz
363Hz
1.0E-7Hz
132Hz
528Hz
10000000Hz
192Hz
293.33333333333Hz
452.57142857143Hz
52.8Hz
264Hz
1320Hz
154Hz
237.6Hz
363Hz
1.0E-7Hz
132Hz
528Hz
10000000Hz

Lambdoma Keyboard (Barbara Hero) colored in with the locally determined frequency , and the letter note values based on a 256Hz C.

	0	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15	16
0	0/0 - -	0/1 - -	0/2 - -	0/3 - -	0/4 - -	0/5 - -	0/6 0Hz zero:f3	0/7 0Hz zero:b3	0/8 - -	0/9 - -	0/10 - -	0/11 - -	0/12 - -	0/13 - -	0/14 - -	0/15 - -	0/16 - -
1	1/0 - -	1/1 - -	1/2 - -	1/3 - -	1/4 - -	1/5 52Hz G#:f10	1/6 44Hz F:b9	1/7 - -	1/8 - -	1/9 - -	1/10 - -	1/11 - -	1/12 - -	1/13 - -	1/14 - -	1/15 - -	1/16 - -
2	2/0 - -	2/1 - -	2/2 - -	2/3 - -	2/4 132Hz C:f4	2/5 105Hz G#:b2	2/6 - -	2/7 - -	2/8 - -	2/9 - -	2/10 - -	2/11 - -	2/12 - -	2/13 - -	2/14 - -	2/15 - -	2/16 - -
3	3/0 - -	3/1 - -	3/2 - -	3/3 264Hz C:f11	3/4 198Hz G:b8	3/5 - -	3/6 - -	3/7 - -	3/8 - -	3/9 - -	3/10 - -	3/11 - -	3/12 - -	3/13 - -	3/14 - -	3/15 - -	3/16 - -
4	4/0 - -	4/1 - -	4/2 528Hz C:f5	4/3 352Hz F:b1	4/4 - -	4/5 - -	4/6 - -	4/7 - -	4/8 - -	4/9 - -	4/10 - -	4/11 - -	4/12 - -	4/13 - -	4/14 - -	4/15 - -	4/16 - -
5	5/0 - -	5/1 1320Hz E:f12	5/2 660Hz E:b7	5/3 - -	5/4 - -	5/5 - -	5/6 - -	5/7 - -	5/8 - -	5/9 - -	5/10 - -	5/11 - -	5/12 - -	5/13 - -	5/14 - -	5/15 - -	5/16 - -
6	6/0 10000000Hz infinity:f6	6/1 1584Hz G:b0	6/2 - -	6/3 - -	6/4 - -	6/5 - -	6/6 - -	6/7 - -	6/8 - -	6/9 - -	6/10 - -	6/11 - -	6/12 - -	6/13 - -	6/14 - -	6/15 - -	6/16 - -
7	7/0 10000000Hz infinity:b6	7/1 - -	7/2 - -	7/3 - -	7/4 - -	7/5 - -	7/6 - -	7/7 - -	7/8 - -	7/9 - -	7/10 - -	7/11 - -	7/12 154Hz Eb:f0	7/13 - -	7/14 - -	7/15 - -	7/16 - -
8	8/0 - -	8/1 - -	8/2 - -	8/3 - -	8/4 - -	8/5 - -	8/6 - -	8/7 - -	8/8 - -	8/9 - -	8/10 - -	8/11 192Hz F#:f7	8/12 176Hz F:b12	8/13 - -	8/14 - -	8/15 - -	8/16 - -
9	9/0 - -	9/1 - -	9/2 - -	9/3 - -	9/4 - -	9/5 - -	9/6 - -	9/7 - -	9/8 - -	9/9 - -	9/10 237Hz Bb:f1	9/11 216Hz A:b5	9/12 - -	9/13 - -	9/14 - -	9/15 - -	9/16 - -
10	10/0 - -	10/1 - -	10/2 - -	10/3 - -	10/4 - -	10/5 - -	10/6 - -	10/7 - -	10/8 - -	10/9 293Hz D:f8	10/10 264Hz C:b11	10/11 - -	10/12 - -	10/13 - -	10/14 - -	10/15 - -	10/16 - -
11	11/0 - -	11/1 - -	11/2 - -	11/3 - -	11/4 - -	11/5 - -	11/6 - -	11/7 - -	11/8 363Hz F#:f2	11/9 322Hz Eb:b4	11/10 - -	11/11 - -	11/12 - -	11/13 - -	11/14 - -	11/15 - -	11/16 - -
12	12/0 - -	12/1 - -	12/2 - -	12/3 - -	12/4 - -	12/5 - -	12/6 - -	12/7 452Hz A:f9	12/8 396Hz G:b10	12/9 - -	12/10 - -	12/11 - -	12/12 - -	12/13 - -	12/14 - -	12/15 - -	12/16 - -
13	13/0 - -	13/1 - -	13/2 - -	13/3 - -	13/4 - -	13/5 - -	13/6 - -	13/7 - -	13/8 - -	13/9 - -	13/10 - -	13/11 - -	13/12 - -	13/13 - -	13/14 - -	13/15 - -	13/16 - -
14	14/0 - -	14/1 - -	14/2 - -	14/3 - -	14/4 - -	14/5 - -	14/6 - -	14/7 - -	14/8 - -	14/9 - -	14/10 - -	14/11 - -	14/12 - -	14/13 - -	14/14 - -	14/15 - -	14/16 - -
15	15/0 - -	15/1 - -	15/2 - -	15/3 - -	15/4 - -	15/5 - -	15/6 - -	15/7 - -	15/8 - -	15/9 - -	15/10 - -	15/11 - -	15/12 - -	15/13 - -	15/14 - -	15/15 - -	15/16 - -
16	16/0 - -	16/1 - -	16/2 - -	16/3 - -	16/4 - -	16/5 - -	16/6 - -	16/7 - -	16/8 - -	16/9 - -	16/10 - -	16/11 - -	16/12 - -	16/13 - -	16/14 - -	16/15 - -	16/16 - -

Right to Left Diagonals across a Row

Forward (Odd/Even) (x,y)|(x+1,y-1)

RL Row 1:	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	E	5+2=7
RL Row 2:	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	G	3+4=7
RL Row 3:	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	F	1+6=7
RL Row 4:	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	G	12+8=20
RL Row 5:	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	C	10+10=20
RL Row 6:	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	F	8+12=20
RL Row 7:	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	G	6+1=7
RL Row 8:	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	F	4+3=7
RL Row 9:	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	G#	2+5=7
RL Row 10:	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	zero	0+7=7
RL Row 11:	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	Eb	11+9=20
RL Row 12:	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	A	9+11=20
RL Row 13:	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	infinity	7+0=7
RL Row 14:	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	E	5+2=7
RL Row 15:	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	G	3+4=7
RL Row 16:	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	F	1+6=7
RL Row 17:	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	G	12+8=20
RL Row 18:	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	C	10+10=20
RL Row 19:	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	F	8+12=20

Order of frequencies: based on a 264Hz baseline or "C"

660Hz
198Hz
44Hz
396Hz
264Hz
176Hz
1584Hz
352Hz
105.6Hz
1.0E-7Hz
322.66666666667Hz
216Hz
10000000Hz
660Hz
198Hz
44Hz
396Hz
264Hz
176Hz

Backward (Odd/Even) (x,y)|(x-1,y+1)

RL Row 1:	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	F#	8+11=19
RL Row 2:	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	D	10+9=19
RL Row 3:	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	A	12+7=19
RL Row 4:	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	G#	1+5=6
RL Row 5:	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	C	3+3=6
RL Row 6:	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	E	5+1=6
RL Row 7:	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	Eb	7+12=19
RL Row 8:	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	Bb	9+10=19
RL Row 9:	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	F#	11+8=19
RL Row 10:	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	zero	0+6=6
RL Row 11:	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	C	2+4=6
RL Row 12:	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	C	4+2=6
RL Row 13:	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	infinity	6+0=6
RL Row 14:	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	F#	8+11=19
RL Row 15:	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	D	10+9=19
RL Row 16:	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	A	12+7=19
RL Row 17:	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	G#	1+5=6
RL Row 18:	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	C	3+3=6
RL Row 19:	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	E	5+1=6

Order of frequencies: based on a 264Hz baseline or "C"

192Hz
293.33333333333Hz
452.57142857143Hz
52.8Hz
264Hz
1320Hz
154Hz
237.6Hz
363Hz
1.0E-7Hz
132Hz
528Hz
10000000Hz
192Hz
293.33333333333Hz
452.57142857143Hz
52.8Hz
264Hz
1320Hz

Lambdoma Keyboard (Barbara Hero) colored in with the locally determined frequency , and the letter note values based on a 256Hz C.

	0	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15	16
0	0/0 - -	0/1 - -	0/2 - -	0/3 - -	0/4 - -	0/5 - -	0/6 0Hz zero:f3	0/7 0Hz zero:b3	0/8 - -	0/9 - -	0/10 - -	0/11 - -	0/12 - -	0/13 - -	0/14 - -	0/15 - -	0/16 - -
1	1/0 - -	1/1 - -	1/2 - -	1/3 - -	1/4 - -	1/5 52Hz G#:f10	1/6 44Hz F:b9	1/7 - -	1/8 - -	1/9 - -	1/10 - -	1/11 - -	1/12 - -	1/13 - -	1/14 - -	1/15 - -	1/16 - -
2	2/0 - -	2/1 - -	2/2 - -	2/3 - -	2/4 132Hz C:f4	2/5 105Hz G#:b2	2/6 - -	2/7 - -	2/8 - -	2/9 - -	2/10 - -	2/11 - -	2/12 - -	2/13 - -	2/14 - -	2/15 - -	2/16 - -
3	3/0 - -	3/1 - -	3/2 - -	3/3 264Hz C:f11	3/4 198Hz G:b8	3/5 - -	3/6 - -	3/7 - -	3/8 - -	3/9 - -	3/10 - -	3/11 - -	3/12 - -	3/13 - -	3/14 - -	3/15 - -	3/16 - -
4	4/0 - -	4/1 - -	4/2 528Hz C:f5	4/3 352Hz F:b1	4/4 - -	4/5 - -	4/6 - -	4/7 - -	4/8 - -	4/9 - -	4/10 - -	4/11 - -	4/12 - -	4/13 - -	4/14 - -	4/15 - -	4/16 - -
5	5/0 - -	5/1 1320Hz E:f12	5/2 660Hz E:b7	5/3 - -	5/4 - -	5/5 - -	5/6 - -	5/7 - -	5/8 - -	5/9 - -	5/10 - -	5/11 - -	5/12 - -	5/13 - -	5/14 - -	5/15 - -	5/16 - -
6	6/0 10000000Hz infinity:f6	6/1 1584Hz G:b0	6/2 - -	6/3 - -	6/4 - -	6/5 - -	6/6 - -	6/7 - -	6/8 - -	6/9 - -	6/10 - -	6/11 - -	6/12 - -	6/13 - -	6/14 - -	6/15 - -	6/16 - -
7	7/0 10000000Hz infinity:b6	7/1 - -	7/2 - -	7/3 - -	7/4 - -	7/5 - -	7/6 - -	7/7 - -	7/8 - -	7/9 - -	7/10 - -	7/11 - -	7/12 154Hz Eb:f0	7/13 - -	7/14 - -	7/15 - -	7/16 - -
8	8/0 - -	8/1 - -	8/2 - -	8/3 - -	8/4 - -	8/5 - -	8/6 - -	8/7 - -	8/8 - -	8/9 - -	8/10 - -	8/11 192Hz F#:f7	8/12 176Hz F:b12	8/13 - -	8/14 - -	8/15 - -	8/16 - -
9	9/0 - -	9/1 - -	9/2 - -	9/3 - -	9/4 - -	9/5 - -	9/6 - -	9/7 - -	9/8 - -	9/9 - -	9/10 237Hz Bb:f1	9/11 216Hz A:b5	9/12 - -	9/13 - -	9/14 - -	9/15 - -	9/16 - -
10	10/0 - -	10/1 - -	10/2 - -	10/3 - -	10/4 - -	10/5 - -	10/6 - -	10/7 - -	10/8 - -	10/9 293Hz D:f8	10/10 264Hz C:b11	10/11 - -	10/12 - -	10/13 - -	10/14 - -	10/15 - -	10/16 - -
11	11/0 - -	11/1 - -	11/2 - -	11/3 - -	11/4 - -	11/5 - -	11/6 - -	11/7 - -	11/8 363Hz F#:f2	11/9 322Hz Eb:b4	11/10 - -	11/11 - -	11/12 - -	11/13 - -	11/14 - -	11/15 - -	11/16 - -
12	12/0 - -	12/1 - -	12/2 - -	12/3 - -	12/4 - -	12/5 - -	12/6 - -	12/7 452Hz A:f9	12/8 396Hz G:b10	12/9 - -	12/10 - -	12/11 - -	12/12 - -	12/13 - -	12/14 - -	12/15 - -	12/16 - -
13	13/0 - -	13/1 - -	13/2 - -	13/3 - -	13/4 - -	13/5 - -	13/6 - -	13/7 - -	13/8 - -	13/9 - -	13/10 - -	13/11 - -	13/12 - -	13/13 - -	13/14 - -	13/15 - -	13/16 - -
14	14/0 - -	14/1 - -	14/2 - -	14/3 - -	14/4 - -	14/5 - -	14/6 - -	14/7 - -	14/8 - -	14/9 - -	14/10 - -	14/11 - -	14/12 - -	14/13 - -	14/14 - -	14/15 - -	14/16 - -
15	15/0 - -	15/1 - -	15/2 - -	15/3 - -	15/4 - -	15/5 - -	15/6 - -	15/7 - -	15/8 - -	15/9 - -	15/10 - -	15/11 - -	15/12 - -	15/13 - -	15/14 - -	15/15 - -	15/16 - -
16	16/0 - -	16/1 - -	16/2 - -	16/3 - -	16/4 - -	16/5 - -	16/6 - -	16/7 - -	16/8 - -	16/9 - -	16/10 - -	16/11 - -	16/12 - -	16/13 - -	16/14 - -	16/15 - -	16/16 - -

Left to Right Diagonals across a Row

Forward (Odd/Even) (x,y)|(x+1,y+1)

LR Row 1:	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	E	5+2=7
LR Row 2:	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	G	3+4=7
LR Row 3:	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	F	1+6=7
LR Row 4:	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	G	12+8=20
LR Row 5:	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	C	10+10=20
LR Row 6:	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	F	8+12=20
LR Row 7:	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	G	6+1=7
LR Row 8:	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	F	4+3=7
LR Row 9:	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	G#	2+5=7
LR Row 10:	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	zero	0+7=7
LR Row 11:	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	Eb	11+9=20
LR Row 12:	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	A	9+11=20
LR Row 13:	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	infinity	7+0=7
LR Row 14:	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	E	5+2=7
LR Row 15:	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	G	3+4=7
LR Row 16:	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	F	1+6=7
LR Row 17:	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	G	12+8=20
LR Row 18:	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	C	10+10=20
LR Row 19:	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	F	8+12=20

Order of frequencies: based on a 264Hz baseline or "C"

660Hz
198Hz
44Hz
396Hz
264Hz
176Hz
1584Hz
352Hz
105.6Hz
1.0E-7Hz
322.66666666667Hz
216Hz
10000000Hz
660Hz
198Hz
44Hz
396Hz
264Hz
176Hz

Backward (Odd/Even) (x,y)|(x-1,y-1)

LR Row 1:	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	F#	8+11=19
LR Row 2:	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	D	10+9=19
LR Row 3:	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	A	12+7=19
LR Row 4:	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	G#	1+5=6
LR Row 5:	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	C	3+3=6
LR Row 6:	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	E	5+1=6
LR Row 7:	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	Eb	7+12=19
LR Row 8:	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	Bb	9+10=19
LR Row 9:	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	F#	11+8=19
LR Row 10:	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	zero	0+6=6
LR Row 11:	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	C	2+4=6
LR Row 12:	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	C	4+2=6
LR Row 13:	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	infinity	6+0=6
LR Row 14:	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	F#	8+11=19
LR Row 15:	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	D	10+9=19
LR Row 16:	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	A	12+7=19
LR Row 17:	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	G#	1+5=6
LR Row 18:	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	C	3+3=6
LR Row 19:	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	E	5+1=6

Order of frequencies: based on a 264Hz baseline or "C"

192Hz
293.33333333333Hz
452.57142857143Hz
52.8Hz
264Hz
1320Hz
154Hz
237.6Hz
363Hz
1.0E-7Hz
132Hz
528Hz
10000000Hz
192Hz
293.33333333333Hz
452.57142857143Hz
52.8Hz
264Hz
1320Hz

Lambdoma Keyboard (Barbara Hero) colored in with the locally determined frequency , and the letter note values based on a 256Hz C.

	0	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15	16
0	0/0 - -	0/1 - -	0/2 - -	0/3 - -	0/4 - -	0/5 - -	0/6 0Hz zero:f3	0/7 0Hz zero:b3	0/8 - -	0/9 - -	0/10 - -	0/11 - -	0/12 - -	0/13 - -	0/14 - -	0/15 - -	0/16 - -
1	1/0 - -	1/1 - -	1/2 - -	1/3 - -	1/4 - -	1/5 52Hz G#:f10	1/6 44Hz F:b9	1/7 - -	1/8 - -	1/9 - -	1/10 - -	1/11 - -	1/12 - -	1/13 - -	1/14 - -	1/15 - -	1/16 - -
2	2/0 - -	2/1 - -	2/2 - -	2/3 - -	2/4 132Hz C:f4	2/5 105Hz G#:b2	2/6 - -	2/7 - -	2/8 - -	2/9 - -	2/10 - -	2/11 - -	2/12 - -	2/13 - -	2/14 - -	2/15 - -	2/16 - -
3	3/0 - -	3/1 - -	3/2 - -	3/3 264Hz C:f11	3/4 198Hz G:b8	3/5 - -	3/6 - -	3/7 - -	3/8 - -	3/9 - -	3/10 - -	3/11 - -	3/12 - -	3/13 - -	3/14 - -	3/15 - -	3/16 - -
4	4/0 - -	4/1 - -	4/2 528Hz C:f5	4/3 352Hz F:b1	4/4 - -	4/5 - -	4/6 - -	4/7 - -	4/8 - -	4/9 - -	4/10 - -	4/11 - -	4/12 - -	4/13 - -	4/14 - -	4/15 - -	4/16 - -
5	5/0 - -	5/1 1320Hz E:f12	5/2 660Hz E:b7	5/3 - -	5/4 - -	5/5 - -	5/6 - -	5/7 - -	5/8 - -	5/9 - -	5/10 - -	5/11 - -	5/12 - -	5/13 - -	5/14 - -	5/15 - -	5/16 - -
6	6/0 10000000Hz infinity:f6	6/1 1584Hz G:b0	6/2 - -	6/3 - -	6/4 - -	6/5 - -	6/6 - -	6/7 - -	6/8 - -	6/9 - -	6/10 - -	6/11 - -	6/12 - -	6/13 - -	6/14 - -	6/15 - -	6/16 - -
7	7/0 10000000Hz infinity:b6	7/1 - -	7/2 - -	7/3 - -	7/4 - -	7/5 - -	7/6 - -	7/7 - -	7/8 - -	7/9 - -	7/10 - -	7/11 - -	7/12 154Hz Eb:f0	7/13 - -	7/14 - -	7/15 - -	7/16 - -
8	8/0 - -	8/1 - -	8/2 - -	8/3 - -	8/4 - -	8/5 - -	8/6 - -	8/7 - -	8/8 - -	8/9 - -	8/10 - -	8/11 192Hz F#:f7	8/12 176Hz F:b12	8/13 - -	8/14 - -	8/15 - -	8/16 - -
9	9/0 - -	9/1 - -	9/2 - -	9/3 - -	9/4 - -	9/5 - -	9/6 - -	9/7 - -	9/8 - -	9/9 - -	9/10 237Hz Bb:f1	9/11 216Hz A:b5	9/12 - -	9/13 - -	9/14 - -	9/15 - -	9/16 - -
10	10/0 - -	10/1 - -	10/2 - -	10/3 - -	10/4 - -	10/5 - -	10/6 - -	10/7 - -	10/8 - -	10/9 293Hz D:f8	10/10 264Hz C:b11	10/11 - -	10/12 - -	10/13 - -	10/14 - -	10/15 - -	10/16 - -
11	11/0 - -	11/1 - -	11/2 - -	11/3 - -	11/4 - -	11/5 - -	11/6 - -	11/7 - -	11/8 363Hz F#:f2	11/9 322Hz Eb:b4	11/10 - -	11/11 - -	11/12 - -	11/13 - -	11/14 - -	11/15 - -	11/16 - -
12	12/0 - -	12/1 - -	12/2 - -	12/3 - -	12/4 - -	12/5 - -	12/6 - -	12/7 452Hz A:f9	12/8 396Hz G:b10	12/9 - -	12/10 - -	12/11 - -	12/12 - -	12/13 - -	12/14 - -	12/15 - -	12/16 - -
13	13/0 - -	13/1 - -	13/2 - -	13/3 - -	13/4 - -	13/5 - -	13/6 - -	13/7 - -	13/8 - -	13/9 - -	13/10 - -	13/11 - -	13/12 - -	13/13 - -	13/14 - -	13/15 - -	13/16 - -
14	14/0 - -	14/1 - -	14/2 - -	14/3 - -	14/4 - -	14/5 - -	14/6 - -	14/7 - -	14/8 - -	14/9 - -	14/10 - -	14/11 - -	14/12 - -	14/13 - -	14/14 - -	14/15 - -	14/16 - -
15	15/0 - -	15/1 - -	15/2 - -	15/3 - -	15/4 - -	15/5 - -	15/6 - -	15/7 - -	15/8 - -	15/9 - -	15/10 - -	15/11 - -	15/12 - -	15/13 - -	15/14 - -	15/15 - -	15/16 - -
16	16/0 - -	16/1 - -	16/2 - -	16/3 - -	16/4 - -	16/5 - -	16/6 - -	16/7 - -	16/8 - -	16/9 - -	16/10 - -	16/11 - -	16/12 - -	16/13 - -	16/14 - -	16/15 - -	16/16 - -

PRIMES: Differences and Harmonics

The bold letters at the end of each row represent the Lambdona Notes that the ratios of repeating increment_prime_original create.

Row

Forward (Primes) (x,y)|(x+1,y)

Row 1:	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	E	5+2=7
Row 2:	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	G	3+4=7
Row 3:	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	F	1+6=7
Row 4:	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	G	12+8=20
Row 5:	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	C	10+10=20
Row 6:	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	F	8+12=20
Row 7:	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	G	6+1=7
Row 8:	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	F	4+3=7
Row 9:	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	G#	2+5=7
Row 10:	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	zero	0+7=7
Row 11:	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	Eb	11+9=20
Row 12:	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	A	9+11=20
Row 13:	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	infinity	7+0=7
Row 14:	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	E	5+2=7
Row 15:	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	G	3+4=7
Row 16:	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	F	1+6=7
Row 17:	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	G	12+8=20
Row 18:	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	C	10+10=20
Row 19:	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	F	8+12=20

Order of frequencies: based on a 20Hz baseline or "C"

660Hz
198Hz
44Hz
396Hz
264Hz
176Hz
1584Hz
352Hz
105.6Hz
1.0E-7Hz
322.66666666667Hz
216Hz
10000000Hz
660Hz
198Hz
44Hz
396Hz
264Hz
176Hz

Backward (Primes) (x,y)|(x-1,y)

Row 1:	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	F#	8+11=19
Row 2:	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	D	10+9=19
Row 3:	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	A	12+7=19
Row 4:	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	G#	1+5=6
Row 5:	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	C	3+3=6
Row 6:	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	E	5+1=6
Row 7:	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	Eb	7+12=19
Row 8:	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	Bb	9+10=19
Row 9:	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	F#	11+8=19
Row 10:	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	zero	0+6=6
Row 11:	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	C	2+4=6
Row 12:	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	C	4+2=6
Row 13:	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	infinity	6+0=6
Row 14:	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	F#	8+11=19
Row 15:	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	D	10+9=19
Row 16:	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	A	12+7=19
Row 17:	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	G#	1+5=6
Row 18:	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	C	3+3=6
Row 19:	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	E	5+1=6

Order of frequencies: based on a 20Hz baseline or "C"

192Hz
293.33333333333Hz
452.57142857143Hz
52.8Hz
264Hz
1320Hz
154Hz
237.6Hz
363Hz
1.0E-7Hz
132Hz
528Hz
10000000Hz
192Hz
293.33333333333Hz
452.57142857143Hz
52.8Hz
264Hz
1320Hz

Lambdoma Keyboard (Barbara Hero) colored in with the locally determined frequency , and the letter note values based on a 256Hz C.

	0	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15	16
0	0/0 - -	0/1 - -	0/2 - -	0/3 - -	0/4 - -	0/5 - -	0/6 0Hz zero:f3	0/7 0Hz zero:b3	0/8 - -	0/9 - -	0/10 - -	0/11 - -	0/12 - -	0/13 - -	0/14 - -	0/15 - -	0/16 - -
1	1/0 - -	1/1 - -	1/2 - -	1/3 - -	1/4 - -	1/5 52Hz G#:f10	1/6 44Hz F:b9	1/7 - -	1/8 - -	1/9 - -	1/10 - -	1/11 - -	1/12 - -	1/13 - -	1/14 - -	1/15 - -	1/16 - -
2	2/0 - -	2/1 - -	2/2 - -	2/3 - -	2/4 132Hz C:f4	2/5 105Hz G#:b2	2/6 - -	2/7 - -	2/8 - -	2/9 - -	2/10 - -	2/11 - -	2/12 - -	2/13 - -	2/14 - -	2/15 - -	2/16 - -
3	3/0 - -	3/1 - -	3/2 - -	3/3 264Hz C:f11	3/4 198Hz G:b8	3/5 - -	3/6 - -	3/7 - -	3/8 - -	3/9 - -	3/10 - -	3/11 - -	3/12 - -	3/13 - -	3/14 - -	3/15 - -	3/16 - -
4	4/0 - -	4/1 - -	4/2 528Hz C:f5	4/3 352Hz F:b1	4/4 - -	4/5 - -	4/6 - -	4/7 - -	4/8 - -	4/9 - -	4/10 - -	4/11 - -	4/12 - -	4/13 - -	4/14 - -	4/15 - -	4/16 - -
5	5/0 - -	5/1 1320Hz E:f12	5/2 660Hz E:b7	5/3 - -	5/4 - -	5/5 - -	5/6 - -	5/7 - -	5/8 - -	5/9 - -	5/10 - -	5/11 - -	5/12 - -	5/13 - -	5/14 - -	5/15 - -	5/16 - -
6	6/0 10000000Hz infinity:f6	6/1 1584Hz G:b0	6/2 - -	6/3 - -	6/4 - -	6/5 - -	6/6 - -	6/7 - -	6/8 - -	6/9 - -	6/10 - -	6/11 - -	6/12 - -	6/13 - -	6/14 - -	6/15 - -	6/16 - -
7	7/0 10000000Hz infinity:b6	7/1 - -	7/2 - -	7/3 - -	7/4 - -	7/5 - -	7/6 - -	7/7 - -	7/8 - -	7/9 - -	7/10 - -	7/11 - -	7/12 154Hz Eb:f0	7/13 - -	7/14 - -	7/15 - -	7/16 - -
8	8/0 - -	8/1 - -	8/2 - -	8/3 - -	8/4 - -	8/5 - -	8/6 - -	8/7 - -	8/8 - -	8/9 - -	8/10 - -	8/11 192Hz F#:f7	8/12 176Hz F:b12	8/13 - -	8/14 - -	8/15 - -	8/16 - -
9	9/0 - -	9/1 - -	9/2 - -	9/3 - -	9/4 - -	9/5 - -	9/6 - -	9/7 - -	9/8 - -	9/9 - -	9/10 237Hz Bb:f1	9/11 216Hz A:b5	9/12 - -	9/13 - -	9/14 - -	9/15 - -	9/16 - -
10	10/0 - -	10/1 - -	10/2 - -	10/3 - -	10/4 - -	10/5 - -	10/6 - -	10/7 - -	10/8 - -	10/9 293Hz D:f8	10/10 264Hz C:b11	10/11 - -	10/12 - -	10/13 - -	10/14 - -	10/15 - -	10/16 - -
11	11/0 - -	11/1 - -	11/2 - -	11/3 - -	11/4 - -	11/5 - -	11/6 - -	11/7 - -	11/8 363Hz F#:f2	11/9 322Hz Eb:b4	11/10 - -	11/11 - -	11/12 - -	11/13 - -	11/14 - -	11/15 - -	11/16 - -
12	12/0 - -	12/1 - -	12/2 - -	12/3 - -	12/4 - -	12/5 - -	12/6 - -	12/7 452Hz A:f9	12/8 396Hz G:b10	12/9 - -	12/10 - -	12/11 - -	12/12 - -	12/13 - -	12/14 - -	12/15 - -	12/16 - -
13	13/0 - -	13/1 - -	13/2 - -	13/3 - -	13/4 - -	13/5 - -	13/6 - -	13/7 - -	13/8 - -	13/9 - -	13/10 - -	13/11 - -	13/12 - -	13/13 - -	13/14 - -	13/15 - -	13/16 - -
14	14/0 - -	14/1 - -	14/2 - -	14/3 - -	14/4 - -	14/5 - -	14/6 - -	14/7 - -	14/8 - -	14/9 - -	14/10 - -	14/11 - -	14/12 - -	14/13 - -	14/14 - -	14/15 - -	14/16 - -
15	15/0 - -	15/1 - -	15/2 - -	15/3 - -	15/4 - -	15/5 - -	15/6 - -	15/7 - -	15/8 - -	15/9 - -	15/10 - -	15/11 - -	15/12 - -	15/13 - -	15/14 - -	15/15 - -	15/16 - -
16	16/0 - -	16/1 - -	16/2 - -	16/3 - -	16/4 - -	16/5 - -	16/6 - -	16/7 - -	16/8 - -	16/9 - -	16/10 - -	16/11 - -	16/12 - -	16/13 - -	16/14 - -	16/15 - -	16/16 - -

Right to Left Diagonals across a Row

Forward (Primes) (x,y)|(x+1,y-1)

RL Row 1:	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	E	5+2=7
RL Row 2:	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	G	3+4=7
RL Row 3:	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	F	1+6=7
RL Row 4:	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	G	12+8=20
RL Row 5:	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	C	10+10=20
RL Row 6:	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	F	8+12=20
RL Row 7:	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	G	6+1=7
RL Row 8:	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	F	4+3=7
RL Row 9:	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	G#	2+5=7
RL Row 10:	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	zero	0+7=7
RL Row 11:	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	Eb	11+9=20
RL Row 12:	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	A	9+11=20
RL Row 13:	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	infinity	7+0=7
RL Row 14:	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	E	5+2=7
RL Row 15:	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	G	3+4=7
RL Row 16:	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	F	1+6=7
RL Row 17:	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	G	12+8=20
RL Row 18:	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	C	10+10=20
RL Row 19:	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	F	8+12=20

Order of frequencies: based on a 20Hz baseline or "C"

660Hz
198Hz
44Hz
396Hz
264Hz
176Hz
1584Hz
352Hz
105.6Hz
1.0E-7Hz
322.66666666667Hz
216Hz
10000000Hz
660Hz
198Hz
44Hz
396Hz
264Hz
176Hz

Backward (Primes) (x,y)|(x-1,y+1)

RL Row 1:	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	F#	8+11=19
RL Row 2:	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	D	10+9=19
RL Row 3:	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	A	12+7=19
RL Row 4:	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	G#	1+5=6
RL Row 5:	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	C	3+3=6
RL Row 6:	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	E	5+1=6
RL Row 7:	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	Eb	7+12=19
RL Row 8:	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	Bb	9+10=19
RL Row 9:	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	F#	11+8=19
RL Row 10:	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	zero	0+6=6
RL Row 11:	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	C	2+4=6
RL Row 12:	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	C	4+2=6
RL Row 13:	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	infinity	6+0=6
RL Row 14:	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	F#	8+11=19
RL Row 15:	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	D	10+9=19
RL Row 16:	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	A	12+7=19
RL Row 17:	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	G#	1+5=6
RL Row 18:	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	C	3+3=6
RL Row 19:	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	E	5+1=6

Order of frequencies: based on a 20Hz baseline or "C"

192Hz
293.33333333333Hz
452.57142857143Hz
52.8Hz
264Hz
1320Hz
154Hz
237.6Hz
363Hz
1.0E-7Hz
132Hz
528Hz
10000000Hz
192Hz
293.33333333333Hz
452.57142857143Hz
52.8Hz
264Hz
1320Hz

Lambdoma Keyboard (Barbara Hero) colored in with the locally determined frequency , and the letter note values based on a 256Hz C.

	0	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15	16
0	0/0 - -	0/1 - -	0/2 - -	0/3 - -	0/4 - -	0/5 - -	0/6 0Hz zero:f3	0/7 0Hz zero:b3	0/8 - -	0/9 - -	0/10 - -	0/11 - -	0/12 - -	0/13 - -	0/14 - -	0/15 - -	0/16 - -
1	1/0 - -	1/1 - -	1/2 - -	1/3 - -	1/4 - -	1/5 52Hz G#:f10	1/6 44Hz F:b9	1/7 - -	1/8 - -	1/9 - -	1/10 - -	1/11 - -	1/12 - -	1/13 - -	1/14 - -	1/15 - -	1/16 - -
2	2/0 - -	2/1 - -	2/2 - -	2/3 - -	2/4 132Hz C:f4	2/5 105Hz G#:b2	2/6 - -	2/7 - -	2/8 - -	2/9 - -	2/10 - -	2/11 - -	2/12 - -	2/13 - -	2/14 - -	2/15 - -	2/16 - -
3	3/0 - -	3/1 - -	3/2 - -	3/3 264Hz C:f11	3/4 198Hz G:b8	3/5 - -	3/6 - -	3/7 - -	3/8 - -	3/9 - -	3/10 - -	3/11 - -	3/12 - -	3/13 - -	3/14 - -	3/15 - -	3/16 - -
4	4/0 - -	4/1 - -	4/2 528Hz C:f5	4/3 352Hz F:b1	4/4 - -	4/5 - -	4/6 - -	4/7 - -	4/8 - -	4/9 - -	4/10 - -	4/11 - -	4/12 - -	4/13 - -	4/14 - -	4/15 - -	4/16 - -
5	5/0 - -	5/1 1320Hz E:f12	5/2 660Hz E:b7	5/3 - -	5/4 - -	5/5 - -	5/6 - -	5/7 - -	5/8 - -	5/9 - -	5/10 - -	5/11 - -	5/12 - -	5/13 - -	5/14 - -	5/15 - -	5/16 - -
6	6/0 10000000Hz infinity:f6	6/1 1584Hz G:b0	6/2 - -	6/3 - -	6/4 - -	6/5 - -	6/6 - -	6/7 - -	6/8 - -	6/9 - -	6/10 - -	6/11 - -	6/12 - -	6/13 - -	6/14 - -	6/15 - -	6/16 - -
7	7/0 10000000Hz infinity:b6	7/1 - -	7/2 - -	7/3 - -	7/4 - -	7/5 - -	7/6 - -	7/7 - -	7/8 - -	7/9 - -	7/10 - -	7/11 - -	7/12 154Hz Eb:f0	7/13 - -	7/14 - -	7/15 - -	7/16 - -
8	8/0 - -	8/1 - -	8/2 - -	8/3 - -	8/4 - -	8/5 - -	8/6 - -	8/7 - -	8/8 - -	8/9 - -	8/10 - -	8/11 192Hz F#:f7	8/12 176Hz F:b12	8/13 - -	8/14 - -	8/15 - -	8/16 - -
9	9/0 - -	9/1 - -	9/2 - -	9/3 - -	9/4 - -	9/5 - -	9/6 - -	9/7 - -	9/8 - -	9/9 - -	9/10 237Hz Bb:f1	9/11 216Hz A:b5	9/12 - -	9/13 - -	9/14 - -	9/15 - -	9/16 - -
10	10/0 - -	10/1 - -	10/2 - -	10/3 - -	10/4 - -	10/5 - -	10/6 - -	10/7 - -	10/8 - -	10/9 293Hz D:f8	10/10 264Hz C:b11	10/11 - -	10/12 - -	10/13 - -	10/14 - -	10/15 - -	10/16 - -
11	11/0 - -	11/1 - -	11/2 - -	11/3 - -	11/4 - -	11/5 - -	11/6 - -	11/7 - -	11/8 363Hz F#:f2	11/9 322Hz Eb:b4	11/10 - -	11/11 - -	11/12 - -	11/13 - -	11/14 - -	11/15 - -	11/16 - -
12	12/0 - -	12/1 - -	12/2 - -	12/3 - -	12/4 - -	12/5 - -	12/6 - -	12/7 452Hz A:f9	12/8 396Hz G:b10	12/9 - -	12/10 - -	12/11 - -	12/12 - -	12/13 - -	12/14 - -	12/15 - -	12/16 - -
13	13/0 - -	13/1 - -	13/2 - -	13/3 - -	13/4 - -	13/5 - -	13/6 - -	13/7 - -	13/8 - -	13/9 - -	13/10 - -	13/11 - -	13/12 - -	13/13 - -	13/14 - -	13/15 - -	13/16 - -
14	14/0 - -	14/1 - -	14/2 - -	14/3 - -	14/4 - -	14/5 - -	14/6 - -	14/7 - -	14/8 - -	14/9 - -	14/10 - -	14/11 - -	14/12 - -	14/13 - -	14/14 - -	14/15 - -	14/16 - -
15	15/0 - -	15/1 - -	15/2 - -	15/3 - -	15/4 - -	15/5 - -	15/6 - -	15/7 - -	15/8 - -	15/9 - -	15/10 - -	15/11 - -	15/12 - -	15/13 - -	15/14 - -	15/15 - -	15/16 - -
16	16/0 - -	16/1 - -	16/2 - -	16/3 - -	16/4 - -	16/5 - -	16/6 - -	16/7 - -	16/8 - -	16/9 - -	16/10 - -	16/11 - -	16/12 - -	16/13 - -	16/14 - -	16/15 - -	16/16 - -

Left to Right Diagonals across a Row

Forward (Odd/Even) (x,y)|(x+1,y+1)

LR Row 1:	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	G	6+1=7
LR Row 2:	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	F	4+3=7
LR Row 3:	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	G#	2+5=7
LR Row 4:	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	zero	0+7=7
LR Row 5:	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	Eb	11+9=20
LR Row 6:	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	A	9+11=20
LR Row 7:	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	infinity	7+0=7
LR Row 8:	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	E	5+2=7
LR Row 9:	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	G	3+4=7
LR Row 10:	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	F	1+6=7
LR Row 11:	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	G	12+8=20
LR Row 12:	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	10	C	10+10=20
LR Row 13:	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	12	8	F	8+12=20
LR Row 14:	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	1	6	G	6+1=7
LR Row 15:	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	3	4	F	4+3=7
LR Row 16:	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	5	2	G#	2+5=7
LR Row 17:	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	zero	0+7=7
LR Row 18:	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	Eb	11+9=20
LR Row 19:	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	11	9	A	9+11=20
LR Row 20:	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	0	7	infinity	7+0=7

Order of frequencies: based on a 20Hz baseline or "C"

1584Hz
352Hz
105.6Hz
1.0E-7Hz
322.66666666667Hz
216Hz
10000000Hz
660Hz
198Hz
44Hz
396Hz
264Hz
176Hz
1584Hz
352Hz
105.6Hz
1.0E-7Hz
322.66666666667Hz
216Hz
10000000Hz

Backward (x,y)|(x-1,y-1)

LR Row 1:	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	Eb	7+12=19
LR Row 2:	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	Bb	9+10=19
LR Row 3:	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	F#	11+8=19
LR Row 4:	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	zero	0+6=6
LR Row 5:	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	C	2+4=6
LR Row 6:	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	C	4+2=6
LR Row 7:	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	infinity	6+0=6
LR Row 8:	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	F#	8+11=19
LR Row 9:	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	D	10+9=19
LR Row 10:	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	A	12+7=19
LR Row 11:	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	G#	1+5=6
LR Row 12:	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	3	C	3+3=6
LR Row 13:	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	1	5	E	5+1=6
LR Row 14:	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	12	7	Eb	7+12=19
LR Row 15:	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	10	9	Bb	9+10=19
LR Row 16:	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	8	11	F#	11+8=19
LR Row 17:	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	zero	0+6=6
LR Row 18:	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	C	2+4=6
LR Row 19:	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	2	4	C	4+2=6
LR Row 20:	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	0	6	infinity	6+0=6

Order of frequencies: based on a 20Hz baseline or "C"

154Hz
237.6Hz
363Hz
1.0E-7Hz
132Hz
528Hz
10000000Hz
192Hz
293.33333333333Hz
452.57142857143Hz
52.8Hz
264Hz
1320Hz
154Hz
237.6Hz
363Hz
1.0E-7Hz
132Hz
528Hz
10000000Hz

Lambdoma Keyboard (Barbara Hero) colored in with the locally determined frequency , and the letter note values based on a 256Hz C.

	0	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15	16
0	0/0 - -	0/1 - -	0/2 - -	0/3 - -	0/4 - -	0/5 - -	0/6 0Hz zero:f3	0/7 0Hz zero:b3	0/8 - -	0/9 - -	0/10 - -	0/11 - -	0/12 - -	0/13 - -	0/14 - -	0/15 - -	0/16 - -
1	1/0 - -	1/1 - -	1/2 - -	1/3 - -	1/4 - -	1/5 52Hz G#:f10	1/6 44Hz F:b9	1/7 - -	1/8 - -	1/9 - -	1/10 - -	1/11 - -	1/12 - -	1/13 - -	1/14 - -	1/15 - -	1/16 - -
2	2/0 - -	2/1 - -	2/2 - -	2/3 - -	2/4 132Hz C:f4	2/5 105Hz G#:b2	2/6 - -	2/7 - -	2/8 - -	2/9 - -	2/10 - -	2/11 - -	2/12 - -	2/13 - -	2/14 - -	2/15 - -	2/16 - -
3	3/0 - -	3/1 - -	3/2 - -	3/3 264Hz C:f11	3/4 198Hz G:b8	3/5 - -	3/6 - -	3/7 - -	3/8 - -	3/9 - -	3/10 - -	3/11 - -	3/12 - -	3/13 - -	3/14 - -	3/15 - -	3/16 - -
4	4/0 - -	4/1 - -	4/2 528Hz C:f5	4/3 352Hz F:b1	4/4 - -	4/5 - -	4/6 - -	4/7 - -	4/8 - -	4/9 - -	4/10 - -	4/11 - -	4/12 - -	4/13 - -	4/14 - -	4/15 - -	4/16 - -
5	5/0 - -	5/1 1320Hz E:f12	5/2 660Hz E:b7	5/3 - -	5/4 - -	5/5 - -	5/6 - -	5/7 - -	5/8 - -	5/9 - -	5/10 - -	5/11 - -	5/12 - -	5/13 - -	5/14 - -	5/15 - -	5/16 - -
6	6/0 10000000Hz infinity:f6	6/1 1584Hz G:b0	6/2 - -	6/3 - -	6/4 - -	6/5 - -	6/6 - -	6/7 - -	6/8 - -	6/9 - -	6/10 - -	6/11 - -	6/12 - -	6/13 - -	6/14 - -	6/15 - -	6/16 - -
7	7/0 10000000Hz infinity:b6	7/1 - -	7/2 - -	7/3 - -	7/4 - -	7/5 - -	7/6 - -	7/7 - -	7/8 - -	7/9 - -	7/10 - -	7/11 - -	7/12 154Hz Eb:f0	7/13 - -	7/14 - -	7/15 - -	7/16 - -
8	8/0 - -	8/1 - -	8/2 - -	8/3 - -	8/4 - -	8/5 - -	8/6 - -	8/7 - -	8/8 - -	8/9 - -	8/10 - -	8/11 192Hz F#:f7	8/12 176Hz F:b12	8/13 - -	8/14 - -	8/15 - -	8/16 - -
9	9/0 - -	9/1 - -	9/2 - -	9/3 - -	9/4 - -	9/5 - -	9/6 - -	9/7 - -	9/8 - -	9/9 - -	9/10 237Hz Bb:f1	9/11 216Hz A:b5	9/12 - -	9/13 - -	9/14 - -	9/15 - -	9/16 - -
10	10/0 - -	10/1 - -	10/2 - -	10/3 - -	10/4 - -	10/5 - -	10/6 - -	10/7 - -	10/8 - -	10/9 293Hz D:f8	10/10 264Hz C:b11	10/11 - -	10/12 - -	10/13 - -	10/14 - -	10/15 - -	10/16 - -
11	11/0 - -	11/1 - -	11/2 - -	11/3 - -	11/4 - -	11/5 - -	11/6 - -	11/7 - -	11/8 363Hz F#:f2	11/9 322Hz Eb:b4	11/10 - -	11/11 - -	11/12 - -	11/13 - -	11/14 - -	11/15 - -	11/16 - -
12	12/0 - -	12/1 - -	12/2 - -	12/3 - -	12/4 - -	12/5 - -	12/6 - -	12/7 452Hz A:f9	12/8 396Hz G:b10	12/9 - -	12/10 - -	12/11 - -	12/12 - -	12/13 - -	12/14 - -	12/15 - -	12/16 - -
13	13/0 - -	13/1 - -	13/2 - -	13/3 - -	13/4 - -	13/5 - -	13/6 - -	13/7 - -	13/8 - -	13/9 - -	13/10 - -	13/11 - -	13/12 - -	13/13 - -	13/14 - -	13/15 - -	13/16 - -
14	14/0 - -	14/1 - -	14/2 - -	14/3 - -	14/4 - -	14/5 - -	14/6 - -	14/7 - -	14/8 - -	14/9 - -	14/10 - -	14/11 - -	14/12 - -	14/13 - -	14/14 - -	14/15 - -	14/16 - -
15	15/0 - -	15/1 - -	15/2 - -	15/3 - -	15/4 - -	15/5 - -	15/6 - -	15/7 - -	15/8 - -	15/9 - -	15/10 - -	15/11 - -	15/12 - -	15/13 - -	15/14 - -	15/15 - -	15/16 - -
16	16/0 - -	16/1 - -	16/2 - -	16/3 - -	16/4 - -	16/5 - -	16/6 - -	16/7 - -	16/8 - -	16/9 - -	16/10 - -	16/11 - -	16/12 - -	16/13 - -	16/14 - -	16/15 - -	16/16 - -

Solving for the 3D: extrapolating the matrix into 3 dimensions creating a fabric that is infinitely scalable in 6 directions: a sample using waveform pairs form the 13/20 matrix:

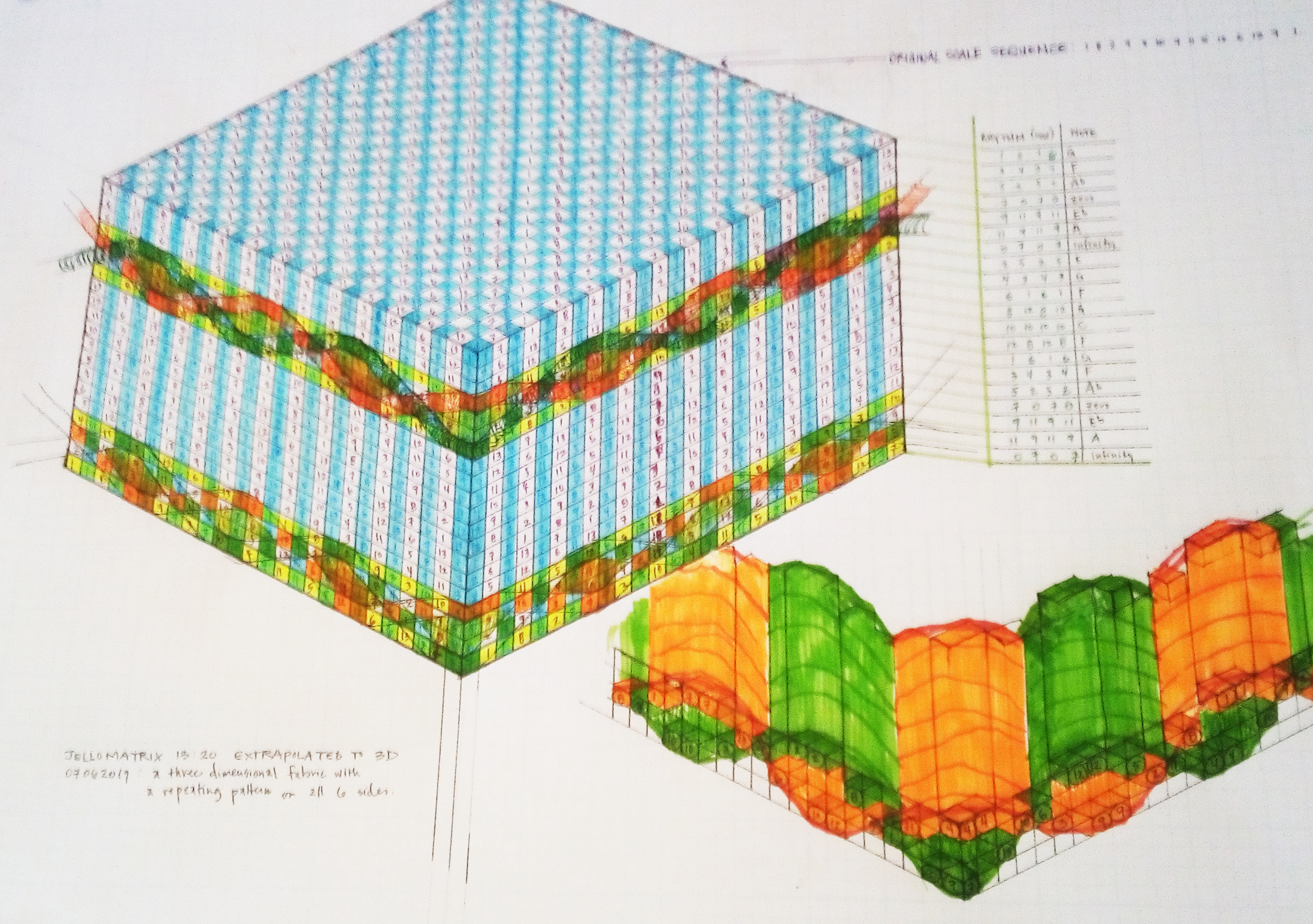
Jellomatrix by Ana Willem is licensed since 2007 under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.
Based on a work at https://www.jellobrain.com.

the skinny.

Obfuscating Calendrical Systems

Here is the result of my argument that the 13/20 based 260 day mayan calendar is actually perhaps another energetically enslaving calendrical system and is perhaps overly complicating things...with 13.20.20.18.20 and all...

I would instead offer the 13/21:
1 year = 365.25 days.
Phi/Fibonacci Ratio (PhiFi) = 13 x 21 = 273
Moon Cycle in days: 27.3 or PhiFi / 10
12 years = 365.25 x 12 = 4,383 days = 273 x 16 = 16 x PhiFi
12 × 15 = 180 years
12 x 180 = 2,160 years
12 x 2160 = 25,920 years = 1 precession each step a factor of 12.

the Emerald Tablet.

In truth, without deceit, certain, and most veritable.

That which is Below corresponds to that which is Above, and that which is Above corresponds to the which is Below, to accomplish the miracles of the One Thing.

And just as all things have come from this One thing, through the mediation of One Mind, so do all created things originate from this One Thing, through Transformation.

It's father is the Sun; it's mother the moon.

The Wind carries it in its belly; its nurse is the Earth.

It is the origin of All, the consecration of the Universe.

Its inherent Strength is perfected, if it is turned into Earth.

Separate the Earth from Fire, the Subtle from the Gross, gently and with great Ingenuity.

It rises from Earth to heaven and descends again to Earth, thereby combining within Itself the powers of both the Above and the Below.

Thus will you obtain the Glory of the Whole Universe. All Obscurity will be clear to you. This is the greatest Force of all powers, because it overcomes every Subtle thing and penetrates every Solid thing.

In this way was the Universe created. From this come many wondrous Applications, because this is the Pattern.

Therefore am I called Thrice Greatest Hermes, having all three parts of the wisdom of the Whole Universe. Herein have I completely explained the Operation of the Sun.

1	8	2	9	3	10	4	11	5	12	6	13	7
2	9	3	10	4	11	5	12	6	13	7	1	8
3	10	4	11	5	12	6	13	7	1	8	2	9
4	11	5	12	6	13	7	1	8	2	9	3	10
5	12	6	13	7	1	8	2	9	3	10	4	11
6	13	7	1	8	2	9	3	10	4	11	5	12
7	1	8	2	9	3	10	4	11	5	12	6	13
8	2	9	3	10	4	11	5	12	6	13	7	1
9	3	10	4	11	5	12	6	13	7	1	8	2
10	4	11	5	12	6	13	7	1	8	2	9	3
11	5	12	6	13	7	1	8	2	9	3	10	4
12	6	13	7	1	8	2	9	3	10	4	11	5
13	7	1	8	2	9	3	10	4	11	5	12	6
1	8	2	9	3	10	4	11	5	12	6	13	7
2	9	3	10	4	11	5	12	6	13	7	1	8
3	10	4	11	5	12	6	13	7	1	8	2	9
4	11	5	12	6	13	7	1	8	2	9	3	10
5	12	6	13	7	1	8	2	9	3	10	4	11
6	13	7	1	8	2	9	3	10	4	11	5	12
7	1	8	2	9	3	10	4	11	5	12	6	13

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	8	2	9	3	10	4	11	5	12	6	13	7
2	9	3	10	4	11	5	12	6	13	7	1	8
3	10	4	11	5	12	6	13	7	1	8	2	9
4	11	5	12	6	13	7	1	8	2	9	3	10
5	12	6	13	7	1	8	2	9	3	10	4	11
6	13	7	1	8	2	9	3	10	4	11	5	12
7	1	8	2	9	3	10	4	11	5	12	6	13
8	2	9	3	10	4	11	5	12	6	13	7	1
9	3	10	4	11	5	12	6	13	7	1	8	2
10	4	11	5	12	6	13	7	1	8	2	9	3
11	5	12	6	13	7	1	8	2	9	3	10	4
12	6	13	7	1	8	2	9	3	10	4	11	5
13	7	1	8	2	9	3	10	4	11	5	12	6
1	8	2	9	3	10	4	11	5	12	6	13	7
2	9	3	10	4	11	5	12	6	13	7	1	8
3	10	4	11	5	12	6	13	7	1	8	2	9
4	11	5	12	6	13	7	1	8	2	9	3	10
5	12	6	13	7	1	8	2	9	3	10	4	11
6	13	7	1	8	2	9	3	10	4	11	5	12
7	1	8	2	9	3	10	4	11	5	12	6	13

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	8	2	9	3	10	4	11	5	12	6	13	7
2	9	3	10	4	11	5	12	6	13	7	1	8
3	10	4	11	5	12	6	13	7	1	8	2	9
4	11	5	12	6	13	7	1	8	2	9	3	10
5	12	6	13	7	1	8	2	9	3	10	4	11
6	13	7	1	8	2	9	3	10	4	11	5	12
7	1	8	2	9	3	10	4	11	5	12	6	13
8	2	9	3	10	4	11	5	12	6	13	7	1
9	3	10	4	11	5	12	6	13	7	1	8	2
10	4	11	5	12	6	13	7	1	8	2	9	3
11	5	12	6	13	7	1	8	2	9	3	10	4
12	6	13	7	1	8	2	9	3	10	4	11	5
13	7	1	8	2	9	3	10	4	11	5	12	6
1	8	2	9	3	10	4	11	5	12	6	13	7
2	9	3	10	4	11	5	12	6	13	7	1	8
3	10	4	11	5	12	6	13	7	1	8	2	9
4	11	5	12	6	13	7	1	8	2	9	3	10
5	12	6	13	7	1	8	2	9	3	10	4	11
6	13	7	1	8	2	9	3	10	4	11	5	12
7	1	8	2	9	3	10	4	11	5	12	6	13

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7

1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7
8	13	2	7	9	1	3	8	10	2	4	9	11	3	5	10	12	4	6	11	13	5	7	12	1	6
9	12	3	6	10	13	4	7	11	1	5	8	12	2	6	9	13	3	7	10	1	4	8	11	2	5
10	11	4	5	11	12	5	6	12	13	6	7	13	1	7	8	1	2	8	9	2	3	9	10	3	4
11	10	5	4	12	11	6	5	13	12	7	6	1	13	8	7	2	1	9	8	3	2	10	9	4	3
12	9	6	3	13	10	7	4	1	11	8	5	2	12	9	6	3	13	10	7	4	1	11	8	5	2
13	8	7	2	1	9	8	3	2	10	9	4	3	11	10	5	4	12	11	6	5	13	12	7	6	1
1	7	8	1	2	8	9	2	3	9	10	3	4	10	11	4	5	11	12	5	6	12	13	6	7	13
2	6	9	13	3	7	10	1	4	8	11	2	5	9	12	3	6	10	13	4	7	11	1	5	8	12
3	5	10	12	4	6	11	13	5	7	12	1	6	8	13	2	7	9	1	3	8	10	2	4	9	11
4	4	11	11	5	5	12	12	6	6	13	13	7	7	1	1	8	8	2	2	9	9	3	3	10	10
5	3	12	10	6	4	13	11	7	5	1	12	8	6	2	13	9	7	3	1	10	8	4	2	11	9
6	2	13	9	7	3	1	10	8	4	2	11	9	5	3	12	10	6	4	13	11	7	5	1	12	8
7	1	1	8	8	2	2	9	9	3	3	10	10	4	4	11	11	5	5	12	12	6	6	13	13	7